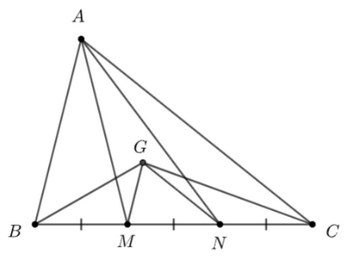
Bài 4.60, 4.61, 4.62, 4.63, 4.64, 4.65 trang 70 SBT Toán 10 tập 1 Kết nối tri thức

B. TỰ LUẬN

Bài 4.60 trang 70 SBT Toán lớp 10 tập 1 - Kết nối tri thức

Trên cạnh BC của tam giác ABC lấy các điểm M, N không trùng với B và C sao cho BM = MN =NC.

a) Chứng minh rằng hai tam giác ABC và AMN có cùng trọng tâm.

b) Gọi \(G\) là trọng tâm của tam giác \(ABC.\) Đặt \(\overrightarrow {GB}  = \overrightarrow u \) và \(\overrightarrow {GC}  = \overrightarrow v .\) hãy biểu thị các vectơ sau qua hai vectơ \(\overrightarrow u \) và \(\overrightarrow v :\,\,\overrightarrow {GA} ,\,\,\overrightarrow {GM} ,\,\,\overrightarrow {GN} .\)

Lời giải:

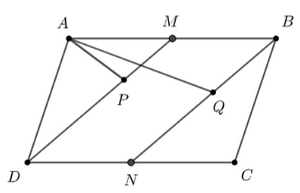
a) Ta có: \(\overrightarrow {AA}  + \overrightarrow {BM}  + \overrightarrow {CN}  = \overrightarrow {BM}  + \overrightarrow {CN}  = \overrightarrow 0 \)

\( \Rightarrow \) hai tam giác \(ABC\) và \(AMN\) có cùng trọng tâm.

b) Gọi \(G\) là trọng tâm của tam giác \(ABC.\) Đặt \(\overrightarrow {GB}  = \overrightarrow u \) và \(\overrightarrow {GC}  = \overrightarrow v .\)

Ta có: \(G\) là trọng tâm của tam giác \(ABC.\)

\( \Rightarrow \) \(\overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC}  = \overrightarrow 0 \)

\( \Rightarrow \) \(\overrightarrow {GA}  =  - \overrightarrow {GB}  - \overrightarrow {GC}  =  - \overrightarrow u  - \overrightarrow v  =  - \left( {\overrightarrow u  + \overrightarrow v } \right)\)

Ta có: \(\overrightarrow {GM}  = \overrightarrow {GB}  + \overrightarrow {BM} \)

\( = \overrightarrow {GB}  + \frac{1}{3}\overrightarrow {BC} \)

\(\begin{array}{l} = \overrightarrow {GB}  + \frac{1}{3}\left( {\overrightarrow {GC}  - \overrightarrow {GB} } \right)\\ = \frac{2}{3}\overrightarrow {GB}  + \frac{1}{3}\overrightarrow {GC}  = \frac{1}{3}\left( {2\overrightarrow u  + \overrightarrow v } \right)\end{array}\)

Ta có: \(\overrightarrow {GN}  = \overrightarrow {GC}  + \overrightarrow {CN} \)

 \(\begin{array}{l} = \overrightarrow {GC}  + \frac{1}{3}\overrightarrow {CB} \\ = \overrightarrow {GC}  + \frac{1}{3}\left( {\overrightarrow {GB}  - \overrightarrow {GC} } \right)\\ = \frac{1}{3}\overrightarrow {GB}  + \frac{2}{3}\overrightarrow {GC}  = \frac{1}{3}\left( {\overrightarrow u  + 2\overrightarrow v } \right)\end{array}\)

Bài 4.61 trang 70 SBT Toán lớp 10 tập 1 - Kết nối tri thức

Cho tam giác \(ABC\) có \(AB = 4,\,\,AC = 5\) và \(\widehat {CAB} = {60^ \circ }.\)

a) Tính tích vô hướng \(\overrightarrow {AB} .\overrightarrow {AC} ,\,\,\overrightarrow {AB} .\overrightarrow {BC} .\)

b) Lấy các điểm \(M,\,\,N\) thỏa mãn \(2\overrightarrow {AM}  + 3\overrightarrow {MC}  = \overrightarrow 0 \) và \(\overrightarrow {NB}  + x\overrightarrow {NC}  = \overrightarrow 0 \,\,\left( {x \ne  - 1} \right).\) Xác định \(x\) sao cho \(AN\) vuông góc với \(BM.\)

Lời giải:

a) Ta có: \(\overrightarrow {AB} .\overrightarrow {AC}  = AB.AC.\cos \widehat {CAB} = 4.5.\cos {60^ \circ } = 10\)

\(\overrightarrow {AB} .\overrightarrow {BC}  = \overrightarrow {AB} \left( {\overrightarrow {AC}  - \overrightarrow {AB} } \right) = \overrightarrow {AB} .\overrightarrow {AC}  - {\overrightarrow {AB} ^2} = 10 - {4^2} =  - 6\)

b) Ta có: \(2\overrightarrow {AM}  + 3\overrightarrow {MC}  = \overrightarrow 0 \)

\( \Leftrightarrow \) \(2\left( {\overrightarrow {AB}  + \overrightarrow {BM} } \right) + 3\left( {\overrightarrow {BC}  - \overrightarrow {BM} } \right) = \overrightarrow 0 \)

\( \Leftrightarrow \) \(\overrightarrow {BM}  =  - 2\overrightarrow {AB}  - 3\overrightarrow {BC}  = 2\overrightarrow {AB}  + 3\left( {\overrightarrow {AC}  - \overrightarrow {AB} } \right) =  - \overrightarrow {AB}  + 3\overrightarrow {AC} \)     (1)

Ta có: \(\overrightarrow {NB}  + x\overrightarrow {NC}  = \overrightarrow 0 \)

\( \Leftrightarrow \) \(\left( {\overrightarrow {AB}  - \overrightarrow {AN} } \right) + x\left( {\overrightarrow {AC}  - \overrightarrow {AN} } \right) = \overrightarrow 0 \)

\( \Leftrightarrow \) \(\left( {1 + x} \right)\overrightarrow {AN}  = \overrightarrow {AB}  + x\overrightarrow {AC} \)            (2)

Từ (1) và (2) \( \Rightarrow \) \(\left( {1 + x} \right)\overrightarrow {AN} .\overrightarrow {BM}  = \left( {\overrightarrow {AB}  + x\overrightarrow {AC} } \right)\left( { - \overrightarrow {AB}  + 3\overrightarrow {AC} } \right)\)

\( \Leftrightarrow \) \(\left( {1 + x} \right)\overrightarrow {AN} .\overrightarrow {BM}  =  - {\overrightarrow {AB} ^2} + 3\overrightarrow {AB} .\overrightarrow {AC}  - x\overrightarrow {AC} .\overrightarrow {AB}  + 3x{\overrightarrow {AC} ^2}\)

\( \Leftrightarrow \) \(\left( {1 + x} \right)\overrightarrow {AN} .\overrightarrow {BM}  =  - 16 + 3.10 - x.10 + 3x.25 = 65x + 14\)

Để \(AN \bot BM\) \( \Leftrightarrow \) \(\overrightarrow {AN} .\overrightarrow {BM}  = 0\)

\( \Leftrightarrow \) \(65x + 14 = 0\) \( \Leftrightarrow \) \(x =  - \frac{{14}}{{64}}\) (thỏa mãn)

Vậy \(x =  - \frac{{14}}{{64}}\) thì \(AN \bot BM\)

Bài 4.62 trang 70 SBT Toán lớp 10 tập 1 - Kết nối tri thức

Cho hình bình hành \(ABCD.\) Gọi \(M,\,\,N\) theo thứ tự là trung điểm các cạnh \(AB,\,\,CD.\) Lấy \(P\) thuộc đoạn \(DM\) và \(Q\) thuộc đoạn \(BN\) sao cho \(DP = 2PM,\,\,BQ = xQN.\) Đặt \(\overrightarrow {AB}  = \overrightarrow u \) và \(\overrightarrow {AD}  = \overrightarrow v .\)

a) Hãy biểu thị các vectơ \(\overrightarrow {AP} ,\,\,\overrightarrow {AQ} \) qua hai vectơ \(\overrightarrow u \) và \(\overrightarrow v .\)

b) Tìm \(x\) để \(A,\,\,P,\,\,Q\) thẳng hàng.

Lời giải:

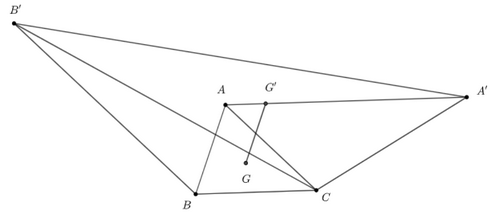
a) Ta có: \(\overrightarrow {AP}  = \overrightarrow {AD}  + \overrightarrow {DP} \)

\(\begin{array}{l} = \overrightarrow {AD}  + \frac{2}{3}\overrightarrow {DM} \\ = \overrightarrow {AD}  + \frac{2}{3}\left( {\overrightarrow {AM}  - \overrightarrow {AD} } \right) = \frac{1}{3}\overrightarrow {AD}  + \frac{2}{3}\overrightarrow {AM} \\ = \frac{1}{3}\overrightarrow {AD}  + \frac{2}{3}.\frac{1}{2}\overrightarrow {AB}  = \frac{1}{3}\overrightarrow u  + \frac{1}{3}\overrightarrow v \end{array}\)

Ta có: \(BQ = xQN\)

\( \Rightarrow \) \(\overrightarrow {BQ}  = x\overrightarrow {QN} \)

\( \Leftrightarrow \) \(\overrightarrow {AQ}  - \overrightarrow {AB}  = x\left( {\overrightarrow {AN}  - \overrightarrow {AQ} } \right)\)

\(\begin{array}{l} \Leftrightarrow \,\,\left( {x + 1} \right)\overrightarrow {AQ}  = \overrightarrow {AB}  + x\overrightarrow {AN} \\ \Leftrightarrow \,\,\left( {x + 1} \right)\overrightarrow {AQ}  = \overrightarrow {AB}  + x\left( {\overrightarrow {AD}  + \overrightarrow {DN} } \right) = x\overrightarrow {AD}  + \overrightarrow {AB}  + x.\frac{1}{2}\overrightarrow {AB} \\ \Leftrightarrow \,\,\left( {x + 1} \right)\overrightarrow {AQ}  = x\overrightarrow {AD}  + \left( {\frac{1}{2}x + 1} \right)\overrightarrow {AB} \\ \Leftrightarrow \,\,\left( {x + 1} \right)\overrightarrow {AQ}  = x\overrightarrow v  + \left( {\frac{1}{2}x + 1} \right)\overrightarrow u \\ \Leftrightarrow \,\,\overrightarrow {AQ}  = \frac{{x + 2}}{{2\left( {x + 1} \right)}}\overrightarrow u  + \frac{x}{{x + 1}}\overrightarrow v \end{array}\)

b) Để \(A,\,\,P,\,\,Q\) thẳng hàng

\( \Leftrightarrow \) \(\overrightarrow {AP} \) và \(\overrightarrow {AQ} \) cùng phương

\( \Leftrightarrow \,\,\frac{{x + 2}}{{2\left( {x + 1} \right)}}:\frac{1}{3} = \frac{x}{{x + 1}}:\frac{1}{3}\) (Điều kiện: \(x \ne  - 1\))

\(\begin{array}{l} \Leftrightarrow \,\,\frac{{x + 2}}{2} = x\\ \Leftrightarrow \,\,2x = x + 2\end{array}\)

\( \Leftrightarrow \,\,x = 2\) (thỏa mãn)

Vậy \(x = 2\) thì \(A,\,\,P,\,\,Q\) thẳng hàng

Bài 4.63 trang 70 SBT Toán lớp 10 tập 1 - Kết nối tri thức

Cho tam giác \(ABC\) với trọng tâm \(G.\) Lấy điểm \(A',\,\,B'\) sao cho \(\overrightarrow {AA'}  = 2\overrightarrow {BC} ,\,\,\overrightarrow {BB'}  = 2\overrightarrow {CA} .\) Gọi \(G'\) là trọng tâm của tam giác \(A'B'C.\) Chứng minh rằng \(GG'\) song song với \(AB.\)

Lời giải:

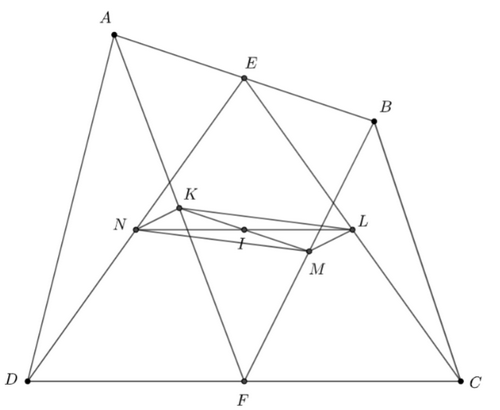
Ta có: \(G\) và \(G'\) lần lượt là trọng tâm của \(\Delta ABC\) và \(\Delta A'B'C\)

\( \Rightarrow \) \(\overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC}  = \overrightarrow 0 \) và \(\overrightarrow {G'A'}  + \overrightarrow {G'B'}  + \overrightarrow {G'C}  = \overrightarrow 0 \)

Ta có: \(\overrightarrow {AA'}  + \overrightarrow {BB'}  + \overrightarrow {CC}  = 3\overrightarrow {GG'} \)

\(\begin{array}{l} \Leftrightarrow \,\,2\overrightarrow {BC}  + 2\overrightarrow {CA}  = 3\overrightarrow {GG'} \\ \Leftrightarrow \,\,2\overrightarrow {BA}  = 3\overrightarrow {GG'} \end{array}\)

\( \Rightarrow \) \(AB\)//\(GG'\) (đpcm)

Bài 4.64 trang 70 SBT Toán lớp 10 tập 1 - Kết nối tri thức

Cho tứ giác lồi \(ABCD,\) không có hai cạnh nào song song. Gọi \(E,\,\,F\) theo thứ tự là trung điểm của \(AB,\,\,CD.\) Gọi \(K,\,\,L,\,\,M,\,\,N\) lần lượt là trung điểm của \(AF,\,\,CE,\,\,BF,\,\,DE.\)

a) Chứng minh rằng tứ giác \(KLMN\) là một hình bình hành.

b) Gọi \(I\) là giao điểm của \(KM,\,\,LN.\) Chứng minh rằng \(E,\,\,I,\,\,F\) thẳng hàng.

Lời giải:

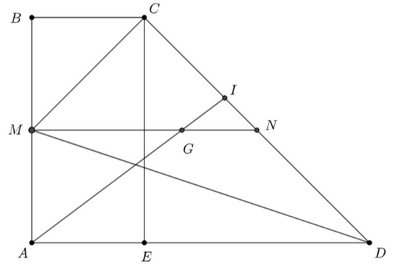
a)      Ta có: \(\overrightarrow {AE}  + \overrightarrow {FC}  = \left( {\overrightarrow {AK}  + \overrightarrow {KL}  + \overrightarrow {LE} } \right) + \left( {\overrightarrow {FK}  + \overrightarrow {KL}  + \overrightarrow {LC} } \right)\)

\( = 2\overrightarrow {KL}  + \left( {\overrightarrow {AK}  + \overrightarrow {FK} } \right) + \left( {\overrightarrow {LE}  + \overrightarrow {LC} } \right)\)

\( = 2\overrightarrow {KL} \)  (1)

Ta có: \(\overrightarrow {EB}  + \overrightarrow {DF}  = \left( {\overrightarrow {EN}  + \overrightarrow {NM}  + \overrightarrow {MB} } \right) + \left( {\overrightarrow {DN}  + \overrightarrow {NM}  + \overrightarrow {MF} } \right)\)

\( = 2\overrightarrow {NM}  + \left( {\overrightarrow {EN}  + \overrightarrow {DN} } \right) + \left( {\overrightarrow {MB}  + \overrightarrow {MF} } \right)\)

\( = 2\overrightarrow {NM} \)  (2)

Ta có: \(\overrightarrow {AE}  + \overrightarrow {FC}  = \overrightarrow {EB}  + \overrightarrow {DF} \) (3)

Từ (1), (2) và (3) \( \Rightarrow \) \(\overrightarrow {KL}  = \overrightarrow {NM} \)

\( \Rightarrow \) tứ giác \(MNKL\) là hình bình hành.

b)  Gọi \(I\) là giao điểm của \(KM,\,\,LN.\)

Ta có: \(\overrightarrow {EI}  = \frac{1}{2}\left( {\overrightarrow {EN}  + \overrightarrow {EL} } \right) = \frac{1}{2}\left( {\frac{1}{2}\overrightarrow {ED}  + \frac{1}{2}\overrightarrow {EC} } \right)\)

\(\begin{array}{l} = \frac{1}{4}\left( {\overrightarrow {ED}  + \overrightarrow {EC} } \right) = \frac{1}{4}.2\overrightarrow {EF} \\ = \frac{1}{2}\overrightarrow {EF} \end{array}\)

\( \Rightarrow \) \(\overrightarrow {EI} \) và \(\overrightarrow {EF} \) cùng hướng

\( \Rightarrow \) ba điểm \(E,\,\,I,\,\,F\) thẳng hàng

Bài 4.65 trang 70 SBT Toán lớp 10 tập 1 - Kết nối tri thức

Cho hình thang vuông \(ABCD\) có \(\widehat {DAB} = \widehat {ABC} = {90^ \circ },\,\,BC = 1,\,\,AB = 2\) và \(AD = 3.\) Gọi \(M\) là trung điểm của \(AB.\)

a) Hãy biểu thị các vectơ \(\overrightarrow {CM} ,\,\,\overrightarrow {CD} \) theo hai vectơ \(\overrightarrow {AB} \) và \(\overrightarrow {AD} .\)

b) Gọi \(N\) là trung điểm của \(CD,\,\,G\) là trọng tâm tam giác \(MCD\) và \(I\) là điểm thuộc cạnh \(CD\) sao cho \(9IC = 5ID.\) Chứng minh rằng \(A,\,\,G,\,\,I\) thẳng hàng.

c) Tính độ dài các đoạn thẳng \(AI\) và \(BI.\)

Lời giải:

a) Ta có: \(BC = 1\) và \(AD = 3\)

mặt khác \(BC\)//\(AD\) vì \(ABCD\) là hình thang vuông tại \(A\) và \(B\)

\( \Rightarrow \) \(\overrightarrow {BC}  = \frac{1}{3}\overrightarrow {AD} \)

Ta có: \(\overrightarrow {CM}  = \overrightarrow {BM}  - \overrightarrow {BC}  = \frac{1}{2}\overrightarrow {AB}  - \frac{1}{3}\overrightarrow {AD} \)

Ta có: \(\overrightarrow {CD}  = \overrightarrow {CB}  + \overrightarrow {BA}  + \overrightarrow {AD} \)

 \(\begin{array}{l} =  - \overrightarrow {BC}  - \overrightarrow {AB}  + \overrightarrow {AD} \\ =  - \frac{1}{3}\overrightarrow {AD}  - \overrightarrow {AB}  + \overrightarrow {AD} \\ = \frac{2}{3}\overrightarrow {AD}  - \overrightarrow {AB} \end{array}\)

b) Ta có: \(G\) là trọng tâm của \(\Delta MCD\)

\( \Rightarrow \) \(3\overrightarrow {AG}  = \overrightarrow {AM}  + \overrightarrow {AC}  + \overrightarrow {AD}  = \frac{1}{2}\overrightarrow {AB}  + \left( {\overrightarrow {AB}  + \overrightarrow {BC} } \right) + \overrightarrow {AD}  = \frac{3}{2}\overrightarrow {AB}  + \frac{4}{3}\overrightarrow {AD} \)

\( \Rightarrow \) \(6.3\overrightarrow {AG}  = 18\overrightarrow {AG}  = 9\overrightarrow {AB}  + 8\overrightarrow {AD} \)           (1)

Ta có: \(9IC = 5ID\)

\( \Rightarrow \) \(9\overrightarrow {IC}  + 5\overrightarrow {ID}  = \overrightarrow 0 \)

\( \Leftrightarrow \) \(9\left( {\overrightarrow {AC}  - \overrightarrow {AI} } \right) + 5\left( {\overrightarrow {AD}  - \overrightarrow {AI} } \right) = \overrightarrow 0 \)

\( \Leftrightarrow \) \(14\overrightarrow {AI}  = 9\overrightarrow {AC}  + 5\overrightarrow {AD} \)

\( \Leftrightarrow \) \(14\overrightarrow {AI}  = 9\left( {\overrightarrow {AB}  + \overrightarrow {BC} } \right) + 5\overrightarrow {AD}  = 9\overrightarrow {AB}  + 9.\frac{1}{3}\overrightarrow {AD}  + 5\overrightarrow {AD} \)

\( \Leftrightarrow \) \(14\overrightarrow {AI}  = 9\overrightarrow {AB}  + 8\overrightarrow {AD} \)                            (2)

Từ (1) và (2) \( \Rightarrow \) \(18\overrightarrow {AG}  = 14\overrightarrow {AI} \)

\( \Rightarrow \) \(\overrightarrow {AG} \) và \(\overrightarrow {AI} \) cùng hướng

\( \Rightarrow \) ba điểm \(A,\,\,G,\,\,I\) thẳng hàng.

c) Ta có: \(14\overrightarrow {AI}  = 9\overrightarrow {AB}  + 8\overrightarrow {AD} \) (cmt)

\( \Rightarrow {\left( {14\overrightarrow {AI} } \right)^2} = {\left( {9\overrightarrow {AB}  + 8\overrightarrow {AD} } \right)^2} = 81{\overrightarrow {AB} ^2} + 144\overrightarrow {AB} .\overrightarrow {AD}  + 64{\overrightarrow {AD} ^2}\)

\( \Rightarrow 194A{I^2} = 81A{B^2} + 64A{D^2} = 81.4 + 64.9 = 900\)

\( \Rightarrow A{I^2} = \frac{{900}}{{196}}\)

\( \Rightarrow AI = \frac{{30}}{{14}} = \frac{{15}}{7}\)

Ta có: \(\overrightarrow {BI}  = \overrightarrow {AI}  - \overrightarrow {AB}  = \frac{9}{{14}}\overrightarrow {AB}  + \frac{4}{7}\overrightarrow {AD}  - \overrightarrow {AB}  = \frac{4}{7}\overrightarrow {AD}  - \frac{5}{{14}}\overrightarrow {AB} \)

\( \Rightarrow \) \(B{I^2} = {\left( {\frac{4}{7}\overrightarrow {AD}  - \frac{5}{{14}}\overrightarrow {AB} } \right)^2} = \frac{{16}}{{49}}{\overrightarrow {AD} ^2} - \frac{{20}}{{49}}\overrightarrow {AD} .\overrightarrow {AB}  + \frac{{25}}{{196}}{\overrightarrow {AB} ^2}\)

\( \Rightarrow \) \(B{I^2} = \frac{{16}}{{49}}{\overrightarrow {AD} ^2} + \frac{{25}}{{196}}{\overrightarrow {AB} ^2} = \frac{{16}}{{49}}.9 + \frac{{25}}{{196}}.4 = \frac{{169}}{{49}}\)

\( \Rightarrow \) \(BI = \frac{{13}}{7}\)

Sachbaitap.com