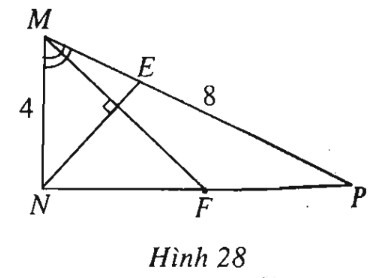
Bài 11 trang 40 SBT Hình học 10 Nâng cao

Tam giác \(MNP\) có \(MN=4, MP=8,\) \(\widehat M = {60^0}\).Lấy điểm \(E\) trên tia \(MP\) và đặt \(\overrightarrow {ME}  = k\overrightarrow {MP} \). Tìm \(k\) để \(NE\) vuông góc với trung tuyến \(MF\) của tam giác \(MNP.\)

Giải

(h.28).

\(\begin{array}{l}\overrightarrow {NE}  = \overrightarrow {NM}  + \overrightarrow {ME}  = k\overrightarrow {MP}  - \overrightarrow {MN},\\\overrightarrow {MF}  = \dfrac{1}{2}(\overrightarrow {MP}  + \overrightarrow {MN} ).\\NE \bot MF\\ \Leftrightarrow(\overrightarrow {MP}  + \overrightarrow {MN} ).(k\overrightarrow {MP}  - \overrightarrow {MN} ) = 0\\\Leftrightarrow \,k = \dfrac{{\overrightarrow {MN} .(\overrightarrow {MP}  + \overrightarrow {MN} )}}{{\overrightarrow {MP} .(\overrightarrow {MP}  + \overrightarrow {MN} )}}\\ = \dfrac{{\overrightarrow {MN} .\overrightarrow {MP}  + {{\overrightarrow {MN} }^2}}}{{{{\overrightarrow {MP} }^2} + \overrightarrow {MN} .\overrightarrow {MP} }}\\ = \dfrac{{16 + 16}}{{64 + 16}} = \dfrac{2}{5}.\end{array}\)

Sachbaitap.com