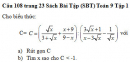Câu 105 trang 23 Sách Bài Tập (SBT) Toán 9 Tập 1Chứng minh các đẳng thức (với a, b không âm và a ≠b) Chứng minh các đẳng thức (với a, b không âm và a ≠b ) a) \({{\sqrt a + \sqrt b } \over {2\sqrt a - 2\sqrt b }} - {{\sqrt a - \sqrt b } \over {2\sqrt a + 2\sqrt b }} - {{2b} \over {b - a}} = {{2\sqrt b } \over {\sqrt a - \sqrt b }}\); b) \(\left( {{{a\sqrt a + b\sqrt b } \over {\sqrt a + \sqrt b }} - \sqrt {ab} } \right){\left( {{{\sqrt a + \sqrt b } \over {a - b}}} \right)^2} = 1.\) Gợi ý làm bài: a) Ta có: \(\eqalign{ (với a, b không âm và a ≠b ) Vế trái bằng vế phải nên đẳng thức được chứng minh. b. Ta có: \(\eqalign{ (với a, b không âm và a ≠b ) Vế trái bằng vế phải nên đẳng thức được chứng minh. Sachbaitap.com
Xem lời giải SGK - Toán 9 - Xem ngay >> Học trực tuyến lớp 9 và Lộ trình UP10 trên Tuyensinh247.com Đầy đủ khoá học các bộ sách: Kết nối tri thức với cuộc sống; Chân trời sáng tạo; Cánh diều. Lộ trình học tập 3 giai đoạn: Học nền tảng lớp 9, Ôn thi vào lớp 10, Luyện Đề. Bứt phá điểm lớp 9, thi vào lớp 10 kết quả cao. Hoàn trả học phí nếu học không hiệu quả. PH/HS tham khảo chi tiết khoá học tại: Link
Xem thêm tại đây:
Ôn tập chương I - Căn bậc hai. Căn bậc ba
|

 Tải ngay
Tải ngay