Câu 5.1 trang 178 sách bài tập Đại số và Giải tích 11 Nâng caoCho hàm số, chứng minh rằng Cho hàm số \(y = \root 3 \of x \) Chứng minh rằng: \(y'\left( x \right) = {1 \over {3\root 3 \of {{x^2}} }}\,\,\left( {x \ne 0} \right)\) Giải Với mỗi \(a \ne 0,\) ta tính đạo hàm của hàm số \(y = \root 3 \of x \) tại điểm theo định nghĩa - Tính \(\Delta y\) \(\Delta y = \root 3 \of {x + \Delta x} - \root 3 \of x \) \(= {{\Delta x} \over {\root 3 \of {{{\left( {x + \Delta x} \right)}^2}} + \root 3 \of {x\left( {x + \Delta x} \right)} + \root 3 \of {{x^2}} }} \) - Tìm giới hạn \(\mathop {\lim }\limits_{\Delta x \to 0} {{\Delta y} \over {\Delta x}} = \mathop {\lim }\limits_{\Delta x \to 0} {1 \over {\root 3 \of {\left( {x + \Delta x} \right)^2} + \root 3 \of {x\left( {x + \Delta x} \right) + \root 3 \of {{x^2}} } }} = {1 \over {3\root 3 \of {{x^2}} }} \) \(= y'\left( x \right)\)
Sachbaitap.com
Xem lời giải SGK - Toán 11 Nâng cao - Xem ngay >> 2K9 Học trực tuyến - Định hướng luyện thi TN THPT, ĐGNL, ĐGTD ngay từ lớp 11 (Xem ngay) cùng thầy cô giáo giỏi trên Tuyensinh247.com. Bứt phá điểm 9,10 chỉ sau 3 tháng, tiếp cận sớm các kì thi.
Xem thêm tại đây:
Bài 1: Khái niệm đạo hàm
|
-

Câu 5.3 trang 179 sách bài tập Đại số và Giải tích 11 Nâng cao
Cho hàm số f (x) = x3 (C) a) Tại những điểm nào của (C) thì tiếp tuyến của (C) có hệ số góc bằng 1.
-

Câu 5.4 trang 179 sách bài tập Đại số và Giải tích 11 Nâng cao
Cho parabol (C) có phương trình y = f (x) = kx2 (k là hằng số khác 0)
-
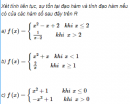
Câu 5.6 trang 179 sách bài tập Đại số và Giải tích 11 Nâng cao
Xét tính liên tục, sự tồn tại đạo hàm và tính đạo hàm nếu có của các hàm số sau đây trên R

 Tải ngay
Tải ngay








